Normal Distribution Tutorial
What do you think the curve’s shape represents? As a data scientist (or aspirant data scientist), you should be able to answer that question at any time. A normal distribution is a concept behind a bell curve, as well as many other applications.
The normal distribution is a fundamental notion in statistics, and it serves as the foundation for data science. We first study the data and try to identify its probability distribution when performing exploratory data analysis, right? And guess what? The Normal Distribution is the most prevalent probability distribution.
Properties
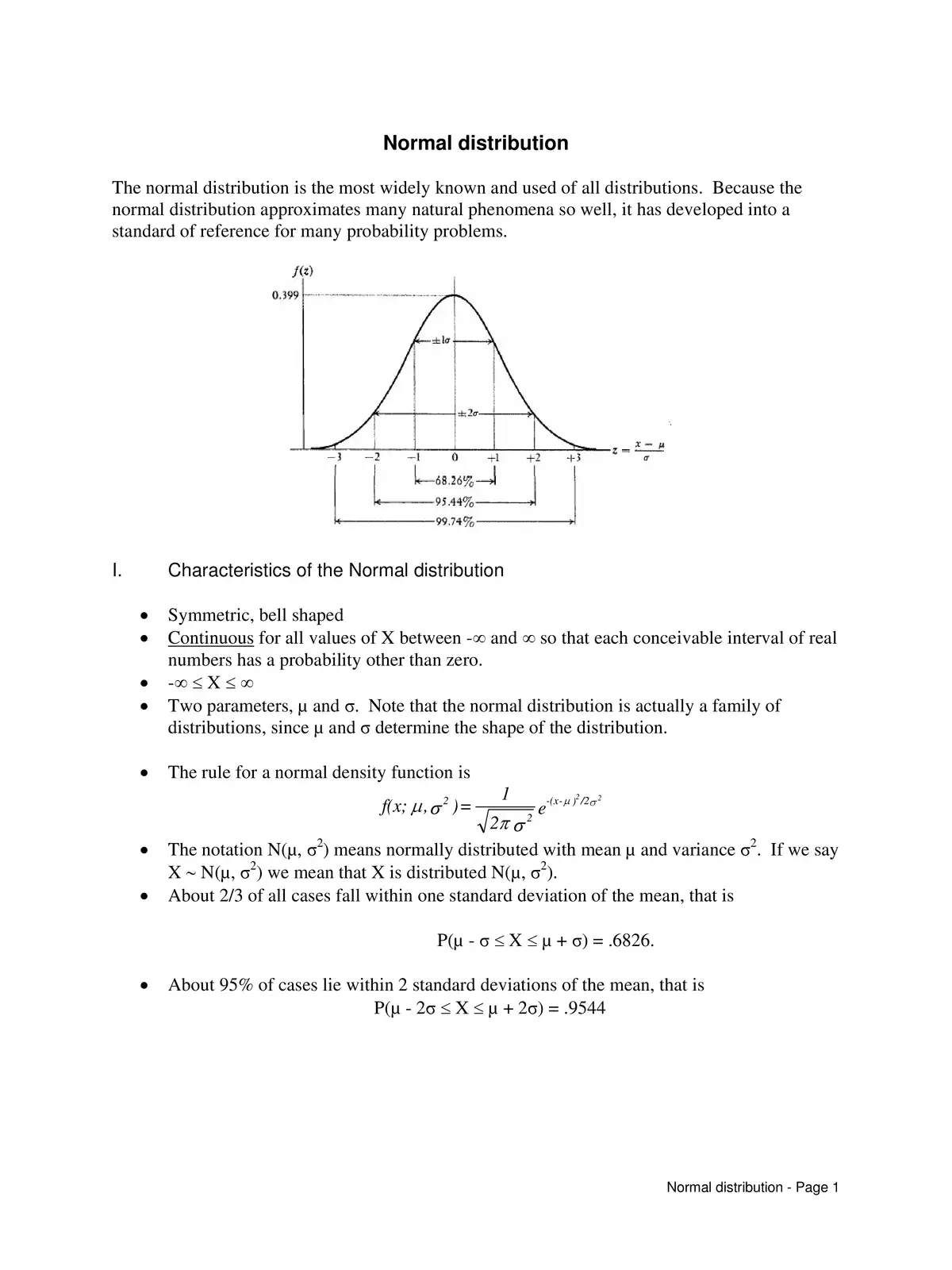
This bell-shaped curve is known as a Normal Distribution. It was discovered by Carl Friedrich Gauss, hence it’s also known as a Gaussian Distribution.
We may simplify the Probability Density of the Normal Distribution by utilizing only two parameters: Mean and 2. Around the Mean, this curve is symmetric. As you can see, the Mean, Median, and Mode for this distribution are all the same.
Note
Another notable feature of a normal distribution is that it maintains its normal shape throughout, unlike other probability distributions, which change their properties after being transformed. For a Normal Distribution, use the following format:
- A Normal Distribution is created by multiplying two Normal Distributions together.
- The Sum of two Normal Distributions is a Normal Distribution.
- A Normal Distribution can be created by converging two Normal Distributions.
- Fourier Transformation of a Normal Distribution is also Normal.
